Stereo Projections: Background, Mathematics, and Use

Interlude: Some Examples

The 3D affine space point P = (-3, 4, 6) is embedded in 4D projective space as P^P = (-3, 4, 6, 1). The following 4D projective space points are projectively equivalent to P^P (and hence also to P):

• (-6, 8, 12, 2)
• (-7.5, 10, 15, 2.5)
• (-6 ³/₇, 8 ⁴/₇, 12 ⁶/₇, 2 ¹/₇)

For each of the following 4D projective space points, to what 3D affine space point do they correspond? That is, to what 3D affine space point are they projectively equivalent?

• (5, 3, 6, 3)
• (-3, 4, 2, 1)
• (-80, 20, 60, 15)

We can characterize all 4D projective space points projectively equivalent to the 3D affine point (x,y,z) as (αx, αy, αz, α) for all non-zero α. Hence to find the affine point corresponding to a given projective space point, simply divide the first three coordinates by the fourth.

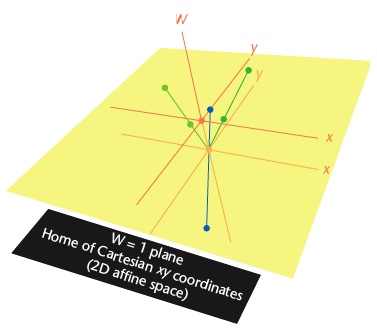
What is the significance of the projective space point (2, -1, -3, 0)? Clearly a line from the projective space origin through this point is parallel to the w=1 plane, so it does not intersect the w=1 plane (our method of determining the corresponding affine point), at least not in a finite distance. It turns out that this can be interpreted as the 3D vector (2, -1, -3).